Back to the Table of Contents

Numbers and Their Application to Math and Science

Odd Solutions for Numbers Lesson 11

Note: all values should be given as exact (in radical form). Decimal approximations are optional, also lend completeness, but must be clearly identified as approximations.

  1. Using the Pythagorean Theorem in its three dimensional form (a2 + b2 + c2 = d2), find and simplify the three face diagonals and the body diagonal of a parallelopiped (box/cuboid) with a = 240, b = 44, c = 117.
    A.
    Face Diagonals: sqrt(2402 + 442) = 244,
    sqrt(442 + 1172) = 125,
    sqrt(2402 + 1172) = 267
    Body diagonal: sqrt(2402 + 442 + 1172) = sqrt(73225) = 5•sqrt(2929)   [approximately equal to] 270.6

  2.  

  3. Find the length of the hypotenuse of a right triangle if the other sides are the same length of 5.
    A. 5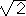

  4.  

  5. Given a 30°-60°-90° triangle with the hypotenuse 14, find the lengths of the other two sides.
    A. 7, 7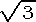

  6.  

  7. Find the distance between the points (-12, 6) and (4, -6).
    A. sqrt[ (-12-4)2 + (6+6)2] = sqrt(162 + 122) = sqrt(400) = 20

  8.  

  9. Driving to Dairy Queen from the MSC, you go 1/4 a mile to the left. The road bends (90°) to the right, and you proceed on for another mile to Main street. At Main Street, you take a left and continue for another 2 miles. Dairy Queen will be on the left side of the road. If you happened to walk directly from MSC to Dairy Queen, how many miles would you save by not driving?
    A. Drawing a right triangle with the hypotenuse as the direct path. The length of one side is 1 mile; the other side is 2 and 1/4 miles. Using Pythagorean Theorem: sqrt[(9/4)2 + 12] = miles walked. sqrt(97/16) [approximately equal to] 2.46 and the miles driven were 3¼. You save about .79 miles.

  10.  

  11. A circle is the set of points equidistant from a given point. If (4,2) is the center with (6,3) on the circle, prove that (2,3) is also on the circle.
    A. The distance between (4,2) and (6,3) is sqrt(5); the distance between (4,2) and (2,3) is sqrt(5). Since (2,3) is the same distance apart, then by definition it is on the circle.

  12.  

  13. Verify rows 3 through 5 of the Fermat-Catalin Conjecture table.
    A.
    Row 3:    73 + 132 = 343 + 169 = 512 = 29 ;       1/3 + 1/2 + 1/9 = 6/18 + 9/18 + 2/18 = 17/18.
    Row 4:   27 + 173 = 128 + 4913 = 5041 = 712;   
    1/7 + 1/3 + 1/2 = 6/42 + 14/42 + 21/42 = 41/42.
    Row 5:   35 + 114 = 243 + 14641 = 14884 = 1222;   
    1/5 + 1/4 + 1/2 = 4/20 + 5/20 + 10/20 = 19/20.

  14.  

  15. Read section 8.6 in your geometry textbook. Do problems 8.6: 12-14, 20-21, 24.
    1.  

    2. {14, 8, 17} = NO because 142 + 82 is not 172

    3.  

    1.  

    2. 82 = 22 + x2 so that the ladder will reach about 7.75 feet on the wall.

    1.  

BACK to the LESSON HOMEWORK ACTIVITY NEXT LESSON